While the primary aim of ecologists is to understand the interactions among organisms and their environment, we also have to be concerned about practical applications in the conservation of ecosystems, the protection of biodiversity, the regulation of composition of the biomass, the management of natural renewable resources – both in space and in time.
Therefore, any positive analysis of an enhancement of the understanding of ecosystems should be accompanied by a normative analysis of identifying possible policies suitable to accomplish a society’s objectives in conservation of the natural environment and sustainable development of mankind.
Optimal Control Theory Can Help Find the Most Suitable Policy Measures
Given a sufficient understanding of the functioning of the ecosystem and the deduced ecological relationships, the formulation of mathematical optimisation problems may help select the most suitable policy measures (from a set of all available policies) to safeguard sustainable development. The mathematical tool that proves particularly helpful in the selection of, in the sense of a given (social) objective function, optimal policies is optimal control theory (OCT). OCT provides a powerful formal framework that can be applied to a vast variety of intertemporal choice problems, as they are typically present in ecological conservation and environmental protection. OCT represents a tool which is very helpful in solving problems with socio-ecological connectivity so that ecologists concerned with those problems may enormously benefit from its application.
OCT, originally developed in engineering, has revolutionized methods in intertemporal optimisation with applications now extending to all areas of human activity. Contrary to its predecessor, the calculus of variations, OCT directly incorporates relevant constraints associated with the problem in a natural way.
To be able to formulate a meaningful mathematical model, OCT presumes that the interactions among organisms and their environment (i.e. the ecological aspects) as well as the co-operation and competition within and between the species under consideration can be quantified in functional relationships; and the same must be true for the available policy measures and their implied consequences. Any problem in OCT is genuinely intertemporal and dynamic such that the stocks of the different species evolve over time, and these processes can be modelled by either difference or differential equations.
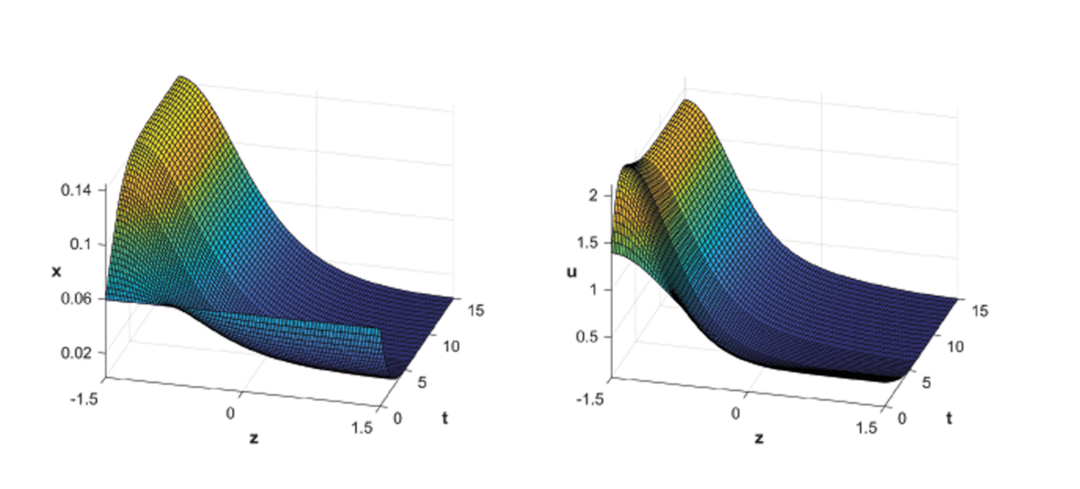
Today’s Decisions Have an Effect on Future Dynamics
The idea of OCT is that an agent, e.g. a regulator, a government, a regulatory agency, firm etc., is able to control those stocks only indirectly by means of policy measures that allow to interfere with their growth conditions. Due to the intertemporal interdependencies any today’s decision has an effect on future states and future dynamics, and thus on the future welfare and on the set of policies available in the future. – And it is this effect on the future that generically renders any intertemporal and in particular any spatio-temporal problem intricate.
Determining Optimal Policy Measures in Time and Space
We are specifically interested in these spatio-temporal ecological-economic problems. In those problems, we not only have to determine how policy measures (e.g. effort, financial resources, conservation measures) should be allocated in time, but also how to distribute them spatially. Examples are the management of fish moving stocks, distributed algae control, harvesting of spatially distributed renewable resources, forest management, sewage disposal, and many other ecological and environmental problems.
» For our research we use Pontryagin‘s maximum principle to find the best possible (optimal) control for taking a dynamical system from one state to another. The maximum principle was formulated in 1956 by the Russian mathematician Lev Pontryagin (1908 – 1988). «
Thorsten Upmann
Beyond these ecological problems, OCT can conveniently be used to identify optimal measures in epidemic control; and specifically – which is now highly topical – in the containment of the transmission and the spread of COVID-19, where the timing and the spatial targeting of cost-effective measures is particularly important. In any of those cases, a government or an agent seeks to manage stocks or the size of the sub-populations so as to either reach a given target at minimal cost or to maximize a given objective function.
While the specific results obtained by OCT differ between models, there is still one important general insight: Even in a completely homogeneous situation (homogeneous space, homogeneous growth dynamics etc.), it may happen that spatially homogeneous policies are not optimal. Specifically, it may be optimal to concentrate protective measures in some areas, leaving others less protected; or to focus activities at specific locations, leaving others subject to less exercise of influence.
